Small Open Economy Extension (IRBC)

Macro II - Fluctuations - ENSAE, 2024-2025

Pablo Winant

2025-03-19

Introduction and Basic Facts

Why a small open economy?

What are the classical reasons to open economy to trade?

  • trade integration
    • taste for variety
    • comparative advantage
  • financial integration
    • smooth shock / insurance

From RBC to IRBC

RBC models have been very successful at matching Business Cycles

  • (temporary) victory against keynesian view that short term fluctuations result from demand shocks
  • so successful that facts at odd with theoretical predictions have been called “puzzles”

It didn’t take long before the same methodology was applied to International Business Cycles

Seminal Paper:

Very successful methodology:

  • facts at odd with theoretical predictions have been called “puzzles”

IRBC Facts

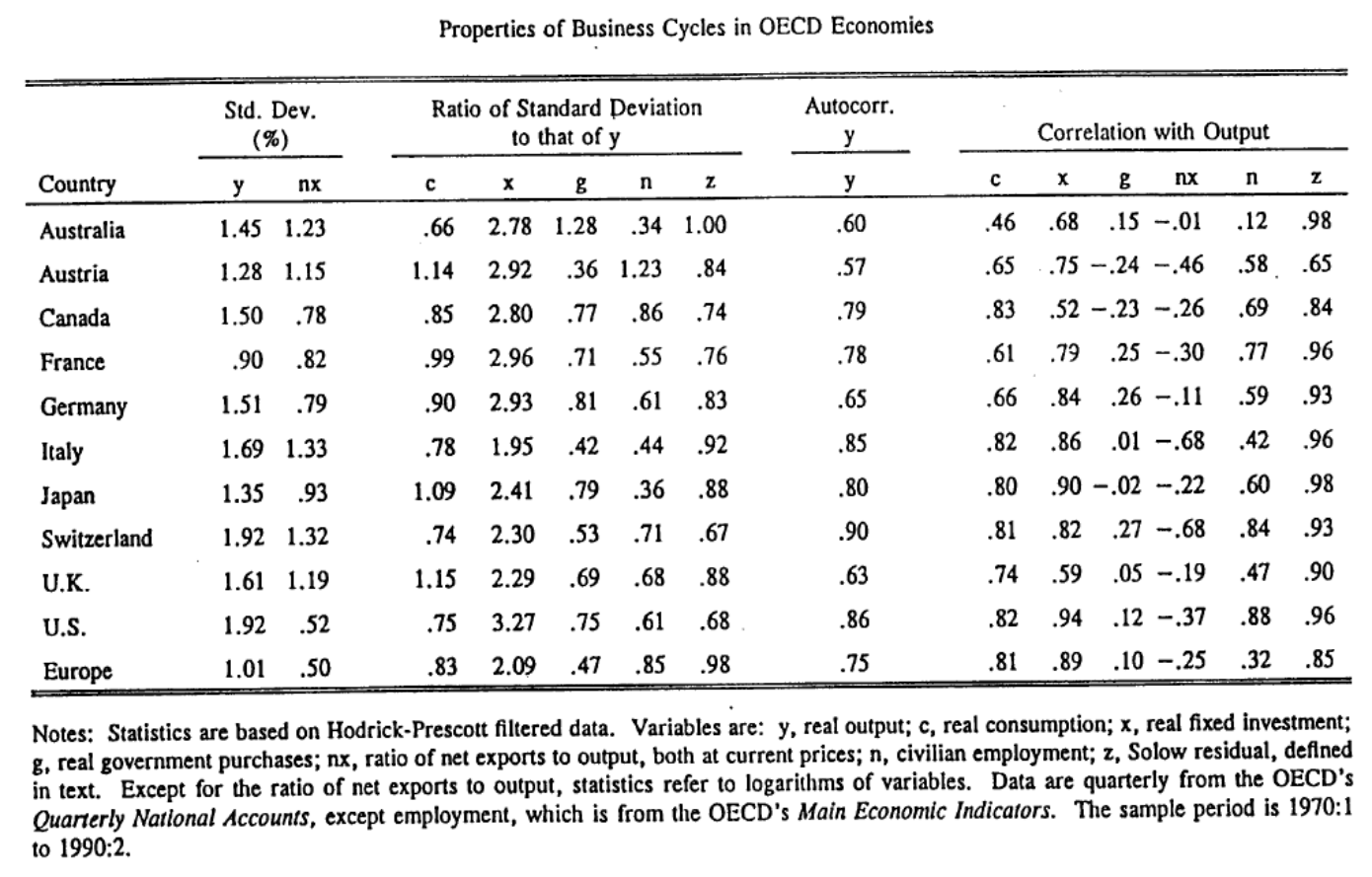
Moments

From Kehoe,Kydland (1995)

IRBC Facts

Moments

Comoments

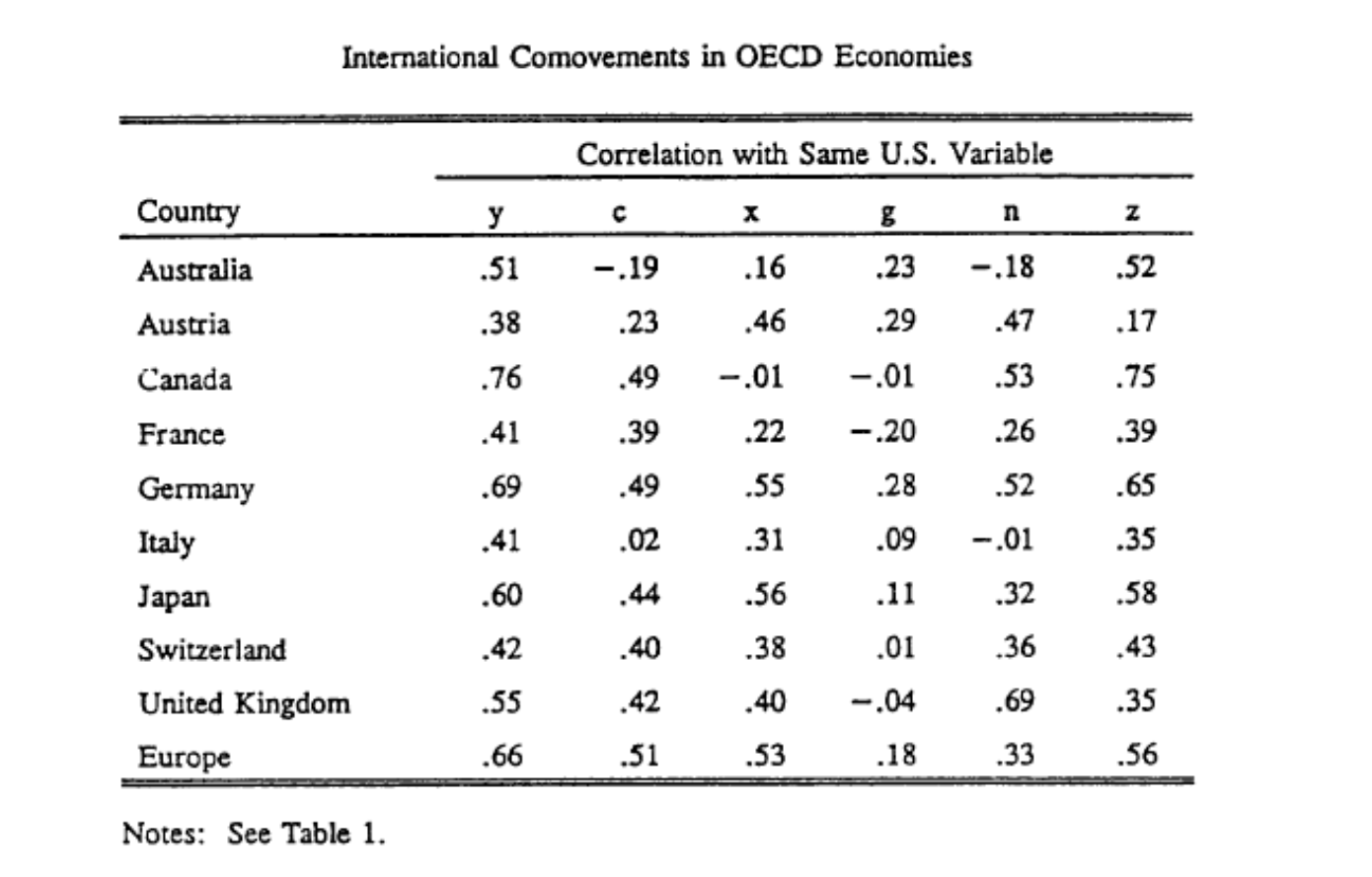
Stylized Facts

Moments

Comoments

Domestically:

  • output more variable than consumption
  • output autocorrelated
  • productivity strongly procyclical
  • trade balance strongly countercyclcal
  • positive comovements in output

Internationally:

  • smaller comovements in consumption
    • Backus-Kehoe-Kydland puzzle

Can we replicate these moments with a BC model?

Modeling a Small Open Economy

Endowment model

Representative agents maximizes: \[\max_{c_t} \sum_{t=0}^{\infty} \beta^t u(c_t)\] \[c_t + a_{t} \leq y_t + (1+r) a_{t-1} \]

Endowment economy:

  • income \((y_t)_t\) is exogenously given
  • for simplicity we assume it is deterministic

Small open economy:

  • open: can save \(a_t\) which yields \(a_{t+1}(1+r)\) in the next period
  • small: country takes world interest rate \(r\) as given (no effect on world prices)

We solve this problem with the terminal conditions:

  • \(a_{-1}\) given1

  • \(\lim_{T\rightarrow\infty} \frac{a_{T}}{(1+r)^T}\geq0\)

    • no-ponzi condition

The no-ponzi condition will in effect eliminate diverging solutions.

In a first order approximation, it selects the right eigenvalues.

Endowment model (3)

We get the lagrangian:

\[\mathcal{L}= \sum_{t=0}^{\infty} \beta^t u(c_t) + \sum_{t=0}^{\infty} \beta^t \lambda_t \left(y_t + (1+r) a_{t-1} - c_t-a_{t} \right)\]

First order conditions:

\[\begin{align} u^{\prime}(c_t)& =& \lambda_t \\ \lambda_t &=& \beta (1+r) \lambda_{t+1} \end{align}\]

Under the technical assumption \(\beta (1+r)=1\) we get \(c_t=c_{t+1}\) then

\[c_0 = \frac{r}{1+r}\left\{ (1+r) a_{-1} + \sum_{t=0}^{\infty} \frac{y_t}{(1+r)^t}\right\}\]

  • consumption is determined by permanent income
    • changes in initial wealth have permanent effects
  • remark: problem isomorphic to consumption-savings decisions

Current Account

Reminders on Current Account

The trade balance is exports-imports (here \(y_t-c_t\))

The current account is trade balance + net factor payments (here \(y_t-c_t+r a_{t-1}\))

Positive current account: additional lending to the rest of the world.

Using the formula from before

\[CA_0 = a_{-1} r + (1-\frac{r}{1+r}) y_0 - \frac{r}{1+r}\left\{ \sum_{t\geq1}^{\infty} \frac{y_t}{(1+r)^t}\right\}\]

How does the current account reacts to income shocks?

  • current account responds positively to temporary shock in income

  • and to news about future income shocks:

    • This is the intertemporal approach to the current account

Unit root

Still with the same formula: \[c_0 = \frac{r}{1+r}\left\{ (1+r) a_{-1} + \sum_{t=0}^{\infty} \frac{y_t}{(1+r)^t}\right\}\]

What is the effect of an increase in \(a_{-1}\)?

- consumption rises permanently
- $a_t$ is constant, equal to $a_{-1}$
- agent consumes small amount $r$ corresponding to interests paid forever on $a_{-1}$
  • this will correspond to a unit root in the solution

Adding capital

We add capital and production to our endowment economy: \[y_t = z_t k_{t-1}^\alpha\] \[k_t = (1-\delta) k_{t-1} + i_{t}\]

The aggregate resource constraint becomes:

\[a_{t} + c_t + i_t = (1+r) a_{t-1} + y_t\]

Now maximize \(\sum_t \beta^ t U(c_t)\)

We get first order conditions

\[\lambda_t = \beta \lambda_{t+1} (1+r)\] \[\lambda_t = \beta \lambda_{t+1}\left[ (1-\delta) + z_{t+1} f^{\prime}(k_{t}) \right]\]

where \(\lambda_t\) is lagrange multiplier associated to budget constraint.

Adding capital: optimality conditions

Since \(\lambda_t>0\) (constraint is always binding), we get:

\[(1-\delta) + z_{t+1} f^{\prime}(k_{t}) = 1+r\]

\[k_{t} = \left( \frac{r+\delta}{\alpha z_{t+1}}\right)^{\frac{1}{\alpha-1}}\]

and investment \[i_t = \left( \frac{r+\delta}{\alpha z_{t+1}}\right)^{\frac{1}{\alpha-1}}- (1-\delta)\left( \frac{r+\delta}{\alpha z_{t}}\right)^{\frac{1}{\alpha-1}}\]

Here investment is fully determined by productivity shocks

  • too simple: no international dependence

Add friction to the investment

A possible solution: change the resource constraint such that adjusting capital is costly

For instance:

\[a_{t} + c_t + i_t + \frac{\omega}{2}\frac{(k_{t}-k_{t-1})^ 2}{k_t} = (1+r)a_{t-1} + z f(k_{t-1})\]

\[k_{t} = (1-\delta) k_{t-1} + i_t\]

where \(\omega\) is an adjustment friction.

Typically, \(\omega\) is chosen so that the model replicates \(\frac{Var(i_t)}{Var(y_t)}\) from the data.

🔜 Cf tutorial.

A benchmark Small Open Economy Model

A benchmark Small Open Economy Model

Stephanie Schmitt Grohe and Martin Uribe

Closing Small Economy Models, Schmitt Grohe and Uribe (2003), JIE

  • small open economy model with production, consumption-leisure tradeoff and capital adjustment costs
    • = RBC+open+adj costs
  • perform some moments matching
  • compare different ways of stationarizing

The model

\[\max_{c_t, n_t} \sum_{t=0}^{\infty} \beta^t u(c_t, n_t)\]

\[c_t + k_{t} + a_{t} = y_t + g_t - \frac{\omega}{2}(k_{t}-k_{t-1})^2 +(1-\delta) k_{t-1} + (1+r^{\star}+{\color{red}\pi(a_{t-1})})a_{t-1}\] \[y_t = f(k_{t-1}, n_t, z_t)\]

\[z_{t+1} = \rho z_t + \epsilon_{t+1}\]

and \[u(c, n) = \frac{1}{1-\sigma}\left(c^{\psi}(1-n)^{1-\psi} )\right)^{1-\sigma}\]

The term \(\color{red}\pi\) is there to make the model stationary.

How to make the distribution stationary?

The solution of the model exhibits a unit root:

\[a_t = a_{t-1} + ... \text{other variables in t-1} + \text{shocks in t}\]

Problem:

  • there isn’t a unique deterministic steady-state
  • the ergodic distribution of the model variables is not defined

This raises practical issues (notably for estimation) for the linear model.

  • no unconditional moments

How to get rid of the unit root?

General idea:

  • introduce a force that pulls the level of foreign assets towards equilibrium

Schmitt Grohe and Uribe (2003) consider many options:

  • debt-elastic interest rate: \[1+r = 1+r^{\star} + \pi(a_d)\]
    • with \(\pi(0)=0\) and \(\pi^{\prime}(0)>0\)
    • \(\pi\) can be understood as a risk premium on rising debt
  • endogenous time-discount (aka Usawa preferences) \[\beta(c_t) = (1+c_t)^{-\chi}\]
  • costs of adjustment for international portfolios

SGU show that the choice of the stationarization device has little effect for the dynamics (moments) of most variables

Calibration

Parameters Values
\(σ\) 2
\(ψ\) 1.45
\(α\) 0.32
\(ω\) 0.028
\(r\) 0.04
Parameters Values
\(δ\) 0.1
\(ρ\) 0.42
\(σ²\) 0.0129
\(A^{\star}\) -0.7442
\(χ\) 0.000742

Results

Impulse Response Function

Moments (from SGU)

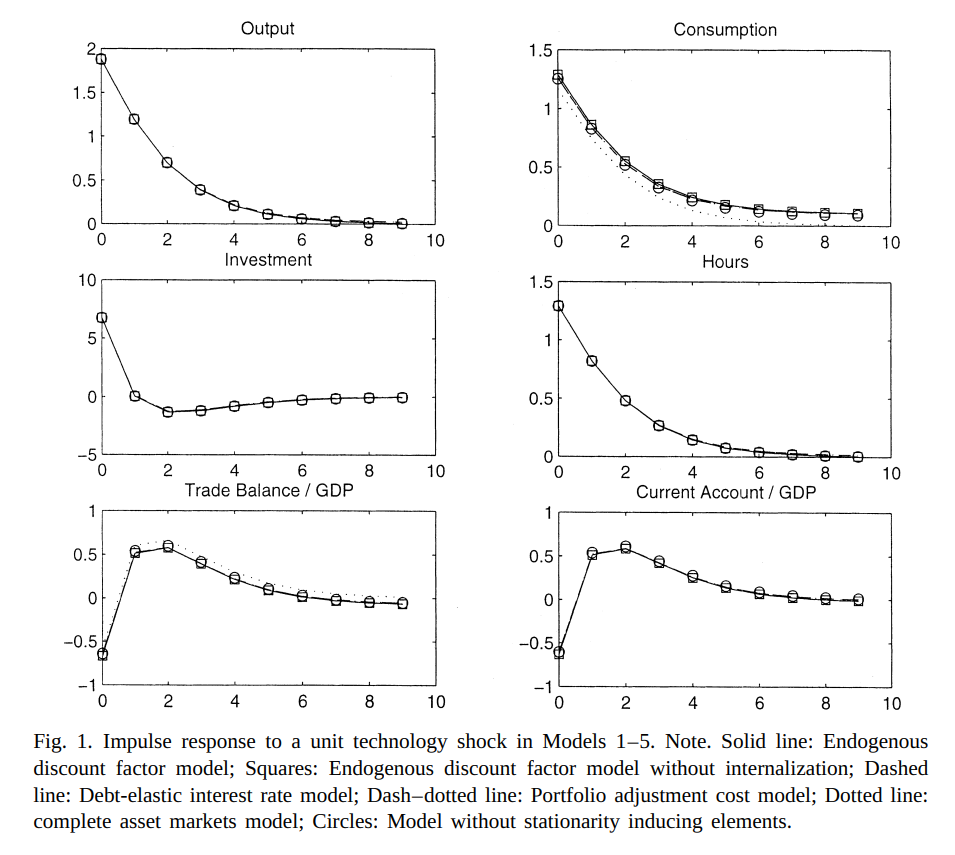
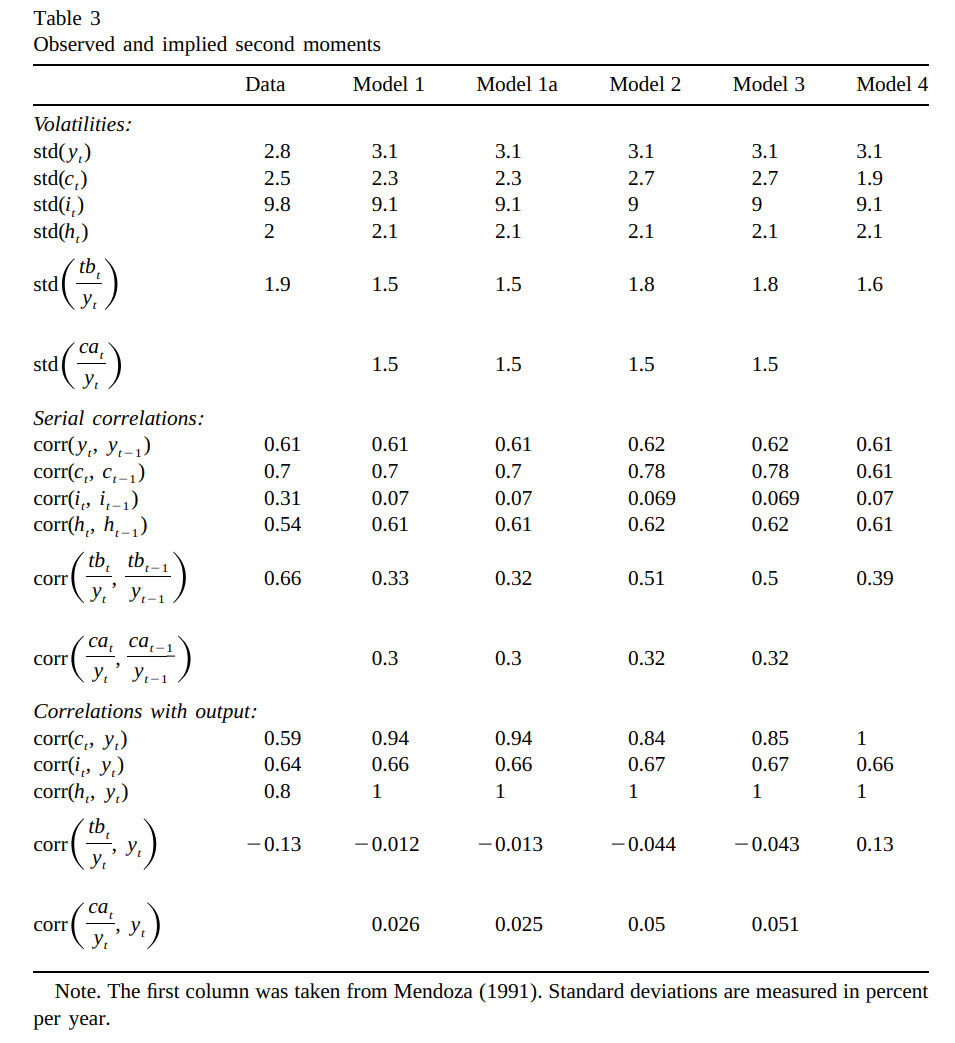
Conclusions

  • The model matches unconditional correlations fairly well
    • The stationarization device has little effect on the moments
  • Unconditional correlations are not that great
    • a limitation of the moment matching method?
  • Correlation of consumption with output is too high
    • and probably cross-correlation of consumption too low
    • still the Backus-Kehoe-Kydland puzzle…